In structural geology, the analysis of rock deformation in the field or at the microscope (strain analysis) allows us making some assumptions on what kind of stresses had caused those deformations (stress analysis).

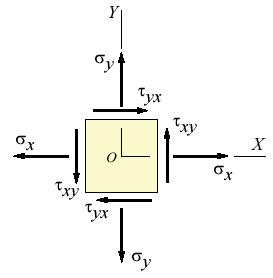
While analyzing stress, it is convenient to describe it within a coordinate system. A 2-dimensional stress has components that are parallel to the x and y axes, that is σ_x e σ_y. The corresponding shear stress will have a component τ_xy (linked to σ_x and parallel to the y axis) and a component τ_yx (linked to σ_y and parallel to the x axis). These are the 4 components of the plane stress (a particular case in which no stress is acting along the z direction, perpendicularly to the xy plane).

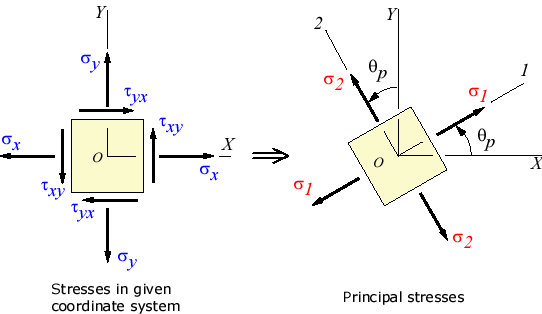
The stress upon a plane oriented by an angle θ with respect to the y axis is represented as in the picture at right. At a certain value of the θ angle, σ will reach its maximum value.

Around 360° there are 2 directions, perpendicular to each other, at which we’ll have a maximum and a minimum value for the shear stress; stresses perpendicular to those surfaces are called the principal stresses σ₁ and σ₂. In these two directions shear stresses are zero.

In three-dimensional stress, the homogeneous stress can always be analyzed in terms of 3 principal stresses perpendicular to each other σ₁ ≥ σ₂ ≥ σ₃, called main, intermediate, and minor principal stress, respectively.

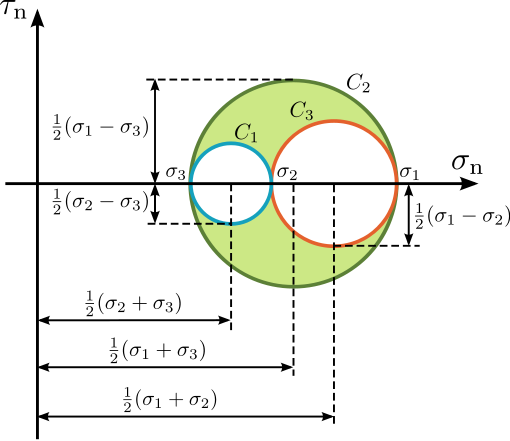
Calculating the stress on a surface whose normal is at a angle θ from the principal main stress σ₁ can be performed on a diagram where σ is the x axis and τ the y axis. All the σ – τ pairs resulting on the different planes while varying the angle θ from 0 to 360° are lying on the so called Mohr’s circle.

The circle intersects the x axis at the values σ₁ and σ₃; its radius is (σ₁ – σ₃)/2; its center is at (σ₁ + σ₃)/2 and corresponds to σ₂.

Maximum and minimum values of the shear stress are always on two planes at the same angle (θ ± 45°) from the main principal stress.

Higher values of shear stress are due to stress differences, not to absolute values of the principal stress. In 3 dimensions, this occurs on two conjugate surfaces which intersect along the intermediate stress axis σ₂ at angles of ± 45° from σ₁ and σ₃.

In different situations from the plane stress, we will have stress components along the z axis. In total, they are the 9 components of the stress tensor, 3 of which are normal to the Cartesian planes: σ_xx, σ_yy and σ_zz.

On planes where the shear stress is zero (τ = 0) they become the principal stress planes σ₁, σ₂ and σ₃.

Stress and strain are “second-order tensors”. A scalar is a zero-order tensor (one value, no directional properties); a vector is a first-order tensor (value and direction). Second-order tensors define the interactions between vectors and directional operators.

There are 3 perpendicular planes upon which no shear stress acts upon. The same goes for deformation, where we talk about principal strain axes.